The interest rate at which cash flows are discounted is referred to as the discount rate.
The equilibrium discount rate is the required rate of return for a particular investment, which means the present value (PV) of the future cash flows discounted at the equilibrium discount rate should be equal to the amount of money invested today. We can also consider the rate as the opportunity cost of current investment. If both the present value (PV) and future value (FV) from n periods from now are known, the discount rate can be solved using the following formula:
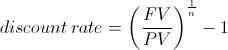
Compounding is the idea that interest is earned not only on the original principal, but also on the interest earned from the previous period. This usually occurs more than once a year. When it is the case, financial institutions usually quote the rate as annual interest rate and compounding frequency instead of quoting the periodic rate. The annual rate of return that investors actually realize is called effective annual rate (EAR). The formula for EAR is:

where:
periodic rate =stated annual rate /m
m =number of compounding periods per year
Example to illustrate EAR:
Assuming the stated annual rate is 10%, if it is compounded annually, this rate will turn $1000 into $1000*(1+10%)=$1100 after one year. If it is compounded monthly, the periodic rate is 10%/12=0.83%. The EAR would be (1+0.83%)12-1 = 10.47%
Using the future value formula, $1000 will grow to $1000 x (1+0.83%)12=$1104.7
In summary, EAR measures the annual rate of return after the adjustments of the compounding periods, therefore EAR is a necessary tool to compare investments that have different compounding periods. For the same stated annual rate, the greater the compounding frequency, the greater EAR will be.
